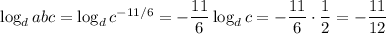Question

If a,b,c and d are positive real numbers such that logab=8/9, logbc=-3/4, logcd=2, find the value of logd(abc)

I really need help on this

Answer 1

We can expand the logarithm of a product as a sum of logarithms:

`\log_dabc=\log_da+\log_db+\log_dc`

Then using the change of base formula, we can derive the relationship

`\log_xy=(\ln y)/(\ln x)=\frac1{(\ln x)/(\ln y)}=\frac1{\log_yx}`

This immediately tells us that

`\log_dc=\frac1{\log_cd}=\frac12`

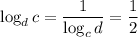
Notice that none of
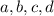`a,b,c,d` can be equal to 1. This is because

`\log_1x=y\implies1^(\log_1x)=1^y\implies x=1`

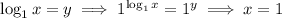
for any choice of
`y`. This means we can safely do the following without worrying about division by 0.

`\log_db=(\ln b)/(\ln d)=((\ln b)/(\ln c))/((\ln d)/(\ln c))=(\log_cb)/(\log_cd)=\frac1{\log_bc\log_cd}`

so that

`\log_db=\frac1{-\frac34\cdot2}=-\frac23`

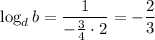
Similarly,

`\log_da=(\ln a)/(\ln d)=((\ln a)/(\ln b))/((\ln d)/(\ln b))=(\log_ba)/(\log_bd)=(\log_db)/(\log_ab)`

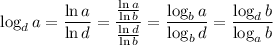
so that

`\log_da=(-\frac23)/(\frac89)=-\frac34`

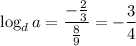
So we end up with

`\log_dabc=-\frac34-\frac23+\frac12=-(11)/(12)`

###

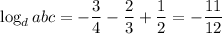
Another way to do this:

`\log_ab=\frac89\implies a^(8/9)=b\implies a=b^(9/8)`

`\log_bc=-\frac34\implies b^(-3/4)=c\implies b=c^(-4/3)`

`\log_cd=2\implies c^2=d\implies\log_dc^2=1\implies\log_dc=\frac12`

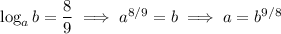
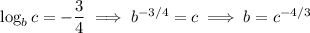
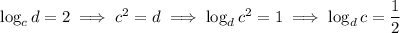
Then

`abc=(c^(-4/3))^(9/8)c^(-4/3)c=c^(-11/6)`

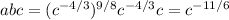
So we have

`\log_dabc=\log_dc^(-11/6)=-\frac{11}6\log_dc=-\frac{11}6\cdot\frac12=-(11)/(12)`