Answer: The length of the hypotenuse is 12√2 units.
Step-by-step explanation: As shown in the attached figure below, the triangle ABC is a right-angled one, where
m∠B = 90°, m∠A = m∠C = 45° and AB = BC = 12 cm.
We are to find the length of the hypotenuse, AC.
From Pythagoras theorem, we have
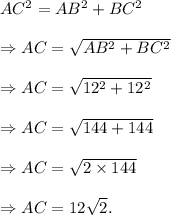
Thus, the length of the hypotenuse is 12√2 units.