Answer:
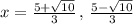
Explanation:
Let
 be a quadratic equation where a , b , c are coefficients and a ≠ 0.
be a quadratic equation where a , b , c are coefficients and a ≠ 0.
Using quadratic formula, roots are given by
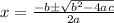
On comparing equation
 with equation
with equation
 , we get
, we get
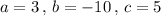
First , we will find
 :
:
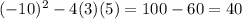
So,
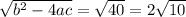
Now, using quadratic formula, we will find roots of the equation .
