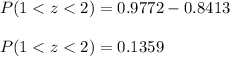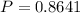Answer:
The area is:
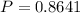
Explanation:
The area under the standard normal curve equals the z probability.
We look for the area of the region that is not shaded.
That is, we look:
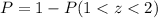
Then we must find
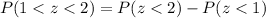
Looking in the standard normal probability table we have to:
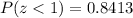
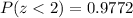
So: