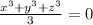Answer:
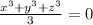
Explanation:
From the question we are told that
mean of three numbers is zero
Generally mean refers to average of number
Let
x, y, z be the three numbers with a mean of zero
T the mean of there cubes
Mathematically the mean of these three numbers is given as

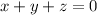
and there cubes
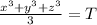

Mathematically solving the above equations by substitution method

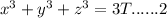
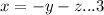
equating 3 in 2
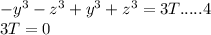
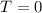
Therefore the mean of the cubes of the three number is 0