Answer:
Question 1)
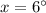
Question 2)
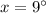
Question 4)
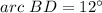
Explanation:
Question 1) we know that
The measure of the interior angle is the semi-sum of the arcs comprising it and its opposite
so
In this problem
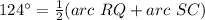
substitute the values and solve for x
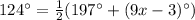
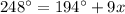


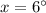
Question 2) we know that
The measurement of the external angle is the semi-difference of the arcs it comprises
so
in this problem
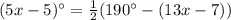
solve for x
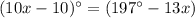
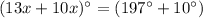
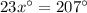
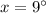
Question 4) we know that
The measurement of the external angle is the semi-difference of the arcs it comprises
so
in this problem
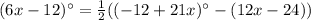
solve for x

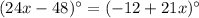
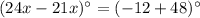

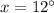
Find the measure of arc BD
