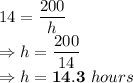Question

Colton is taking a long bike ride where he rides at a constant speed. The distance he bikes is proportional to the amount of time he has been biking. After 3.5 hours, he has traveled a distance of 49 miles.(b) Colton travels at the same rate as in (a) for 6 hours. Let d be the distance he biked. Set up and solve a proportion for d. (c) Colton would like to bike 200 miles. Let h be the number of hours it will take Colton to bike this distance. Solve a proportion for h. Round to the nearest tenth of an hour.

Answer 1

b)
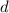`d` = 84 miles

c)
`h` = 14.3 hours

Explanation:

Given that:

The speed of Colton is constant while riding a bike.

Formula for speed is given as:

`Speed = (Distance)/(Time)`

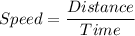
If speed is constant, then we can deduct that:

`Distance \propto Time`

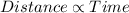
Time = 3.5 hours

Distance = 49 miles

Therefore, the speed will be equal to:

Speed =
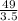`(49)/(3.5)` = 14 miles/hr

b) Given that:

Time = 6 hours, then we have to find the distance to be hiked if the speed is same.

`14 = (d)/(6)\\\Rightarrow d = \bold{84}\ miles/hr`

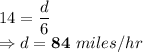
c) Given that:

Distance = 200 miles

Number of hours =
`h`

To find, the value of
`h`.

`14 = (200)/(h)\\\Rightarrow h = (200)/(14)\\\Rightarrow h = \bold{14.3}\ hours`