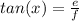Question

What is the tangent of angle x in this rectangle?

Answer 1

`tan (x) = \frac {e} {f}`

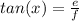
Explanation:

We are given a rectangle within which are two right angles triangles and we are to find the tangent of angle x.

According to the properties of rectangle, the length and width for the both the sides are same so for the triangle with angle x, our perpendicular is
`e`, base is
`f` and hypotenuse is
`f`.

To find the tangent of x, we can use tan:

`tan (x) = \frac {e} {f}`

Answer 2

Hello!

The answer is:
`tan(x)=(e)/(f)`

Why?

Since there is a rectangle, it means that there are two right triangles, we can use the second triangle dimensions in order to find the tangent of the angle "x".

`tan(x)=(Opposite)/(Adjacent)= (e)/(f)`

Where:

`e=Opposite\\f=Adjacent\\g=Hypotenuse`

We can also determine the sine and cosine of the angle "x"

`sin(x)=(Opposite)/(Hypotenuse)=(e)/(g)\\\\cos(x)=(Adjacent)/(Hypotenuse)=(f)/(g)`

Have a nice day!