Answer:
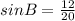
Explanation:
We are given a right angled triangle ABC with known side lengths 16 and 20. We are to find the value of sin B.
We know that sine is the ratio of perpendicular to hypotenuse. But here, the side length for perpendicular is unknown so firstly, we will find AC.
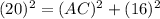
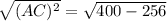
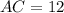
Therefore,
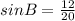 .
.