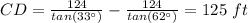Answer:
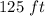
Explanation:
see the attached figure to better understand the problem
step 1
In the right triangle ABC find the length side BC
we know that
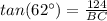
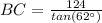
step 2
In the right triangle ABD find the length side BD
we know that
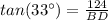
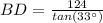
step 3
we know that
The distance between the two boats is the length side CD
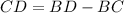
substitute the values