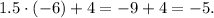Answer:
D
Explanation:
A linear function has an expression
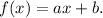 If the set of coordinates {(0, 4), (-2, 1), (-4, -2)} defines a linear function, then
If the set of coordinates {(0, 4), (-2, 1), (-4, -2)} defines a linear function, then
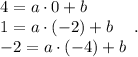
From the first equation,
 Substitute it into the second equation:
Substitute it into the second equation:
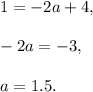
Check it with the third equation:
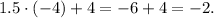
Hence the linear function is
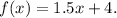
Now check all options:
A.
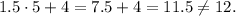
B.

C.
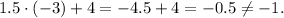
D.