Question

-3x6y4/-3x6y4 in simplest form

Answer 1

1.

Explanation:

-3x6y4/-3x6y4

The top of the fraction is exactly the same as the bottom

So the answer is 1.

Answer 2

For this case we must simplify the following expression:

`\frac {-3x ^ 6y ^ 4} {- 3x ^ 6y ^ 4} =`

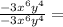
The signs "-" are canceled, then:

`\frac {3x ^ 6y ^ 4} {3x ^ 6y ^ 4} =`

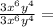
In this case, it is observed that we have a unitary fraction, which by definition is that which has the numerator equal to the denominator.

Example:

`\frac {a} {a} = 1`

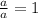
So:

`\frac {3x ^ 6y ^ 4} {3x ^ 6y ^ 4} = 1`

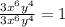
Answer:

1