Answer:
The correct choice is C
Explanation:
The given function is:
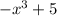
The degree of this function is odd so the function will rise at one end and fall on the other end.
Since the coefficient of the leading term is negative, the graph of the function will rise at the left and fall on the right.
The correct answer is option C.