Question

Which conic section does the equation below describe?

x^2+y^2- 8x+ 10y+15 = 0
O A. Hyperbola
O B. Parabola
OC. Circle
OD. Elipse

Answer 1

`\large\boxed{\boxed{\pink {\bf \leadsto The \ equation \ represents \ a \ circle. }}}`

Explanation:

Given equation to us is ,

`\green{\implies x^2 + y^2 - 8x + 10y + 15 = 0 }`

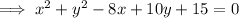
And we need to find which conic section does the equation below describe . So for that let's simpify the Equation .

`\implies x^2 + y^2 - 8x + 10y + 15 = 0 \\\\\implies x^2 - 8x + y^2 + 10y = (-15) \\\\ \implies x^2 -8x + 4^2 - 4^2 + y^2 + 10y + 5^2-5^2 + 15 = 0\\\\ \implies (x -4)^2 + (y+5)^2 - 16 - 25 + 15 = 0 \\\\ \implies (x -4)^2 + (y+5)^2 - 26 = 0 \\\\\implies (x-4)^2 + (y+5)^2 = 26 \\\\\implies (x-4)^2+(y+5)^2=(\sqrt26)^2`

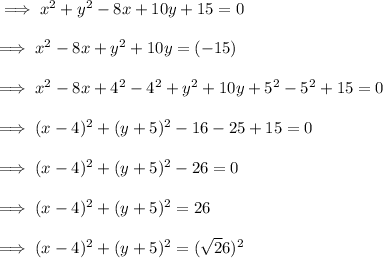
And this is similar to the standard equation for a circle is ( x - h )² + ( y - k )²= r² , where ( h, k ) is the center and r is the radius.

Hence the given equation represents a circle with center ( 4 , -5) and radius √26 units.