There are no solutions to this system. If
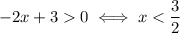
the line is positive and the exponential is negative, so they can't have any point in common.
At
 , the derivative of the line is
, the derivative of the line is
 , whereas the derivative of the exponential is
, whereas the derivative of the exponential is
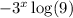 .
.
Since
 for all
for all
 , there can't be other solutions because the exponential is already below the line, and it decreases at a faster rate.
, there can't be other solutions because the exponential is already below the line, and it decreases at a faster rate.