Question

Rutherfordium- 265 is a radioactive substance that has a half-life of 13 hours. If there is a 149g sample of Rutherfordium- 265 left at 12am on October 19th, approximately how much Rutherfordium- 265 would remain on October 22nd at 12am?

Answer 1

3.21 grams

Explanation:

First we are finding the radioactive decay constant using the formula:

lambda =
`(ln(2))/(half-life)`

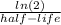
where

lambda is the radioactive decay constant.

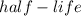`half-life` is the half life of the radioactive substance.

We know from our problem that Rutherfordium- 265 has a half-life of 13 hours, so let's replace the value in our formula.

lambda =
`(ln(2))/(13hours)`

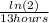
lambda = 0.0533 per hour

Now we can use the decay formula to find the remaining quantity of the substance:

`N_t=N_0e^{(-lamda)(t)`

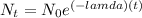
where

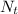`N_t` is the ending amount

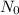`N_0` is the beginning amount

`t` is the time (in hours)

We know from our problem that there is a 149g sample of Rutherfordium- 265 left at 12am on October 19th, so
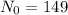`N_0=149`. Notice that there are exactly 3 days from 12 am October 19th to 12 am October 22nd, so we have
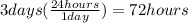`3days((24hours)/(1day) )=72hours`; therefore
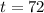`t=72`. Now we can replace all the values in our formula:

`N_t=149e^{(-0.0533)(72)`

`N_t=3.21`

We can conclude that 3.21 grams of Rutherfordium- 265 would remain on October 22nd at 12 am.