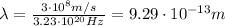1. Frequency:
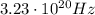
The energy given is the energy per mole of particles:
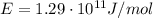
1 mole contains a number of Avogadro of particles,
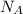 , equal to
, equal to
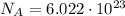 particles
particles
So, by setting the following proportion, we can calculate the energy of a single photon:

This is the energy of a single photon; now we can calculate its frequency by using the formula:
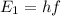
where
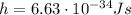 is the Planck's constant
is the Planck's constant
f is the photon frequency
Solving for f, we find
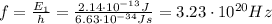
2. Wavelength:
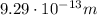
The wavelength of the photon is given by the equation:
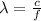
where
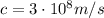
is the speed of the photon (the speed of light). Substituting,