Question

PLEASE HELP ME AS SOON AS POSSIBLE

Answer 1

`f^-^1(x)=(x-9)^2+4` OR
`x^2-18x+85` if you simplify it

Step-by-step explanation:

to find the inverse function you have to switch x and y with each other and solve for y.

`y=9+√(x-4)\\x=9+√(y-4)` step 1: switch x and y with each other

`x-9=√(y-4)` step 2: subtract 9 from both sides

`(x-9)^2=√(y-4)^2` step 3: square both sides to get rid of the square root

`(x-9)^2=y-4\\(x-9)^2+4=y\\` step 4: add 4 to both sides

you could leave the answer like this or you can simplify to get
`x^2-18x+85`

Answer 2

Answer:
`f^(-1)(\text{x}) = (\text{x}-9)^2+4` when
`\text{x} \ge 9`

=================================================

Work Shown:

`f(\text{x}) = 9 + \sqrt{\text{x} - 4}\\\\\text{y} = 9 + \sqrt{\text{x} - 4}\\\\\text{x} = 9 + \sqrt{\text{y} - 4}\\\\\text{x}-9 = \sqrt{\text{y} - 4}\\\\(\text{x}-9)^2 = (\sqrt{\text{y} - 4})^2\\\\(\text{x}-9)^2 = \text{y}-4\\\\(\text{x}-9)^2+4 = \text{y}\\\\f^(-1)(x) = (\text{x} - 9)^2+4`

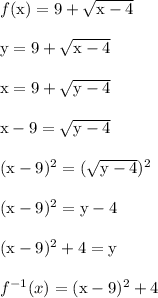
Step-by-step explanation:

I replaced f(x) with y. After that I swapped x and y, then solved for y to get the inverse.

The smallest that
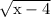`\sqrt{\text{x}-4}` can get is 0, which means the smallest f(x) can get is 9+0 = 9. The range for f(x) is
`\text{y} \ge 9`

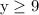
Since x and y swap to determine the inverse, the domain and range swap roles. Therefore, the domain of the inverse
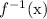`f^(-1)(\text{x})` is
`\text{x} \ge 9`

So we will only consider the right half portion of the parabola.

The graph is below. The red curve mirrors over the black dashed line to get the blue curve, and vice versa.