Question

Which monomial is a perfect cube?

1x3


3x3


6x3


9x3

Answer 1

Option A is correct

`1x^3`

Explanation:

Perfect cube is a number that is the cube of any integer number.

For example:

`7^3 = 343`

`(ab)^3 = a^3b^3`

From the given options we have to find which monomial is a perfect cube.

Consider the monomial
`1x^3`.

We can write 1 as:

`1 = 1 \cdot 1 \cdot 1 = 1^3`

It can be written as:

`1x^3 = 1^3 \cdot x^3 = (1x)^3`

Therefore,
`1x^3` monomial is a perfect cube

Answer 2

Option (a) is correct.

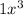`1x^3` is a perfect cube.

Explanation:

Given : Some Monomial

We have to choose out of given monomial that is a perfect cube.

Perfect cubes are numbers that can be written as
`(ab)^3`

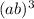
So, consider
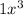`1x^3` , we know 1 can be written as
`1^3`

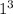
Thus,
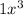`1x^3` can be written as
`1^3x^3=(1x)^3`

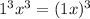
Thus, Option (a) is correct.