Question

Please help me with this

Answer 1

QUESTION 1i)

The given equation passes through

(3,11) and (-2,1).

The slope of the line is

`m = (11 - 1)/(3 - - 2)`

`m = (10)/(5)=2`

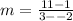
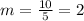
The equation is given by

`y-y_1=m(x-x_1)`

We can use any of the given points.

`y - 1 = 2(x - - 2)`

`y = 2(x + 2) + 1`

`y = 2x + 4 + 1`

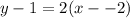
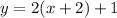
The equation is

`y = 2x + 5`

QUESTION 1ii)

A point that lies on this line must satisfy the equation of this line.

We can choose any value for x and substitute to find the corresponding y-value.

When x=0,

`y = 2(0) + 5 = 5`

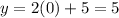
Therefore, the point (0,5) lies on this line.

QUESTION 1iii)

The point (0,6) does not lie on this line.

We can verify this by substituting (0,6) into the equation of the line.

`6 = 2(0) + 5`

`6 = 5`

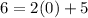
This statement is false.

QUESTION 2

The line passes through, (0,4) and (2,0).

The slope of this line is

`m = (4 - 0)/(0 - 2) = - 2`

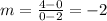
The equation of this line is

`y - 0 = - 2(x - 2)`

`y = - 2x + 4`

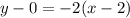
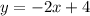
We substitute the point (-25,81) to determine whether this point lies on this line.

`81 = - 2( - 25) + 4`

`81 = 104`

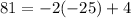
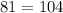
This is a false statement.

The point (-25,81) does not lie on this line.