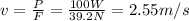Answer:
2.55 m/s
Step-by-step explanation:
The power output can be rewritten as:
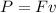 (1)
(1)
where
F is the force applied
v is the speed at which the bucket is raised
If we want to raise the bucket at constant speed, we must apply a force equal and opposite to the weight of the bucket, which is equal to the product between its mass (4.00 kg) and the gravitational acceleration (9.8 m/s^2):

We know that the power output is P = 100 W, so we can re-arrange eq.(1) to find the speed: