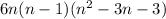Answer:
The factored form is :
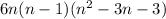
Explanation:
The given expression is :
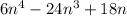
Taking out 6n out as 6n is common for all, we get
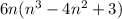
Now lets factor
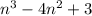 by hit and trial method.
by hit and trial method.
Putting n=1
Now, by hit and trial method, we put n=1,
p(n)=

=> p(1) =
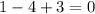
So, (n-1) is a factor.
Now, dividing
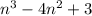 by n-1 we get
by n-1 we get
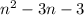
Therefore, the factored form is =