Question

Which algebraic equation best represents Jarrett's hair growth rate? David's hair grows at a rate four times as fast as Jarrett's. A) D= 1 4 B) D= 4 J C) J=D·4 D) J= 1 4 ·D

Answer 1

The answer is D

Explanation:

Answer 2

D.
`J=(1)/(4)D`

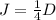
Explanation:

Let D represent David's hair growth and J represent Jarrett's hair growth.

We have been given that David's hair grows at a rate four times as fast as Jarrett's. We are asked to find the algebraic equation that best represents Jarrett's hair growth rate.

We can represent our given information in an equation as:

`D=4*J`

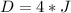
Dividing both sides by 4 we will get,

`(D)/(4)=(4*J)/(4)`

`(1)/(4)D=J`

`J=(1)/(4)D`

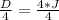
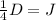
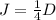
Therefore, the equation
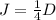`J=(1)/(4)D` best represents Jarrett's hair growth rate and option D is the correct choice.