Question

The ice cream shop offers 31 flavors. you order a double scoop cone how many different ways can the clerk put the ice cream on the cone if you want two different flavors​

Answer 1

465

Explanation:

Since we are looking for the the number of combinations to have 2 different flavors in the ice cream, we can use the formula for combination.

`_(n)C_(k)=(n!)/(k!(n-k)!)`

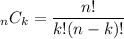
Our variables are:

n = 31

k = 2

`_(31)C_(2)=(31!)/(2!(31-2)!)`

`_(31)C_(2)=(31!)/(2!29!)`

`_(31)C_(2)=(31!)/(2!29!)`

`_(31)C_(2)=465`

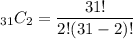
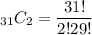
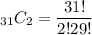
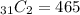
The number of possible combinations of having 2 different flavors out of 31 different flavors is 465.