Question

The isotope calcium-41 decays into potassium-41, with a half-life of 1.03 × 10^5 years. There is a sample of calcium-41 containing 5 × 10^9 atoms. How many atoms of calcium-41 and potassium-41 will there be after 4.12 × 10^5 years? How many calcium and how many potassium?​

Answer 1

1.) Find The Number of Half Lives Passed:

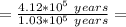`= (4.12*10^(5) \ years)/(1.03*10^(5) \ years) =` half lives

2.) Calculate Number of Ca Atoms remaining:

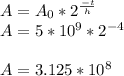`A = A_(0) *2^{(-t)/(h)}\\A = 5*10^9 *2^(-4)\\\\A = 3.125*10^8` atoms left

3. Calculate Number of Potassium Atoms

Subtract the final number of Calcium atoms from the initial amount of calcium atoms.

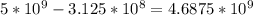`5*10^9 - 3.125*10^8 = 4.6875*10^9` Atoms of Potassium