Answer: The correct options are
(B) 6, 18, 54, 162, 486, . . .
(D) -4,,-2, -1, -0.5, -0.25, -0.125, . . .
Step-by-step explanation: We are given to select all the sequences that are geometric.
We know that a sequence <a(n)> is geometric is there exists a common ratio r such that
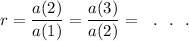
Option (A) :
Here, the given sequence is
2, 5, 8, 11, 14, 17, . . .
We see that
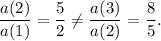
So, this sequence is not geometric and the option (A) is not correct.
Option (B) :
Here, the given sequence is
6, 18, 54, 1562, 486, . . .
We see that
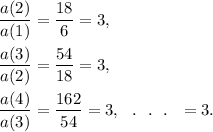
So,
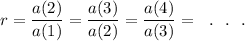
Therefore, the sequence is a geometric sequence and so the option (B) is CORRECT.
Option (C) :
Here, the given sequence is
2, 3, 5, 8, 13, 21, . . .
We see that
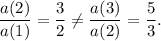
So, this sequence is not geometric and the option (C) is not correct.
Option (D) :
Here, the given sequence is
-4, -2, -1, -0.5, -0.25, -0.125, . . .
We see that
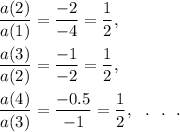
So,
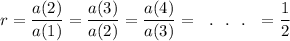
Therefore, the sequence is a geometric sequence and so the option (B) is CORRECT.
Thus, (B) and (D) are the correct options.