Answer:
Choice C is correct.
Explanation:
We have given the expression :
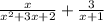
We have to find the sum of terms.
First, we have to find the LCM of the expression.
The LCM is x²+3x+2
The factorization of this term is :
(x+2)(x+1)
(x)+(3)(x+2)/(x+2)(x+1)
(x+3x+6)/(x+2)(x+1)
(4x+6)/(x+2)(x+1)
The nominator of simplified sum is (4x+6).