Question

An object 25.4-centimeters high is located 91.5 centimeters from a concave mirror, focal length 15 centimeters.

A) Determine location of image.

B) Determine the height of the image.


please answer

Answer 1

A) 17.9 cm

We have:

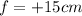`f=+15 cm` is the focal length of the mirror (for concave mirror, the focal length is positive)

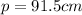`p=91.5 cm` is the distance between the object and the mirror

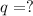`q=?` is the distance between the image and the mirror

We can then use the mirror equation to find q:

`(1)/(f)=(1)/(p)+(1)/(q)\\(1)/(q)=(1)/(f)-(1)/(p)=(1)/(15 cm)-(1)/(91.5 cm)=0.056 cm^(-1)\\q=(1)/(0.056 cm^(-1))=17.9 cm`

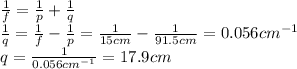
B) -5.0 cm

The height of the image can be determined by using the magnification equation:

`(q)/(p)=-(h_i)/(h_o)`

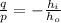
where

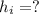`h_i = ?` is the heigth of the image

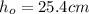`h_o = 25.4 cm` is the height of the object

Substituting and re-arranging the formula, we find

`h_i = -h_o (q)/(p)=-(25.4 cm)(17.9 cm)/(91.5 cm)=-5.0 cm`

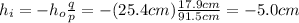
And the negative sign means the image is inverted.