Answer:
No, tyler is wrong, his circle´s area has four times the Lin’s circle area.
Explanation:
Hello
Let's remember this about a circle
the area is given by:
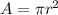
where r is the radius
and the diameter
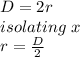
Step 1
according to the question Tyler’s circle has twice the diameter of Lin’s circle,in other terms
Let
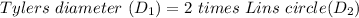

Step 2
find the areas
Area of Tyler’s circle
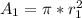
replacing
let r_{1}= Tyler’s circle radius
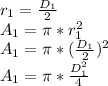
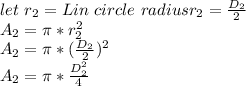
Now, from

replacing
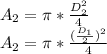
step 3
compare the areas
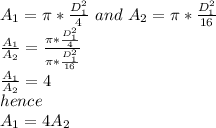
this means that tyler is wrong, his circle´s area has four times the Lin’s circle area.
Have a great day