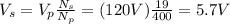Question

1. A step-up transformer increases 15.7V to 110V. What is the current in the secondary as compared to the primary? Assume 100 percent efficiency.2. An ideal step-down transformer has a primary coil of 400 turns and a secondary coil of 19 turns. It's primary coil is plugged into an outlet with 120V (AC), from which it draws an rms current of 0.51A.What is the voltage in the secondary coil?

Answer 1

1.
`I_2 = 0.14 I_1`

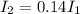
Step-by-step explanation:

We have:

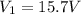`V_1 = 15.7 V` voltage in the primary coil

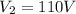`V_2 = 110 V` voltage in the secondary coil

The efficiency of the transformer is 100%: this means that the power in the primary coil and in the secondary coil are equal

`P_1 = P_2\\V_1 I_1 = V_2 I_2`

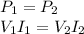
where I1 and I2 are the currents in the two coils. Re-arranging the equation, we find

`(I_2)/(I_1)=(V_1)/(V_2)=(15.7 V)/(110 V)=0.14`

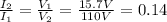
which means that the current in the secondary coil is 14% of the value of the current in the primary coil.

2. 5.7 V

We can solve the problem by using the transformer equation:

`(N_p)/(N_s)=(V_p)/(V_s)`

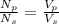
where:

Np = 400 is the number of turns in the primary coil

Ns = 19 is the number of turns in the secondary coil

Vp = 120 V is the voltage in the primary coil

Vs = ? is the voltage in the secondary coil

Re-arranging the formula and substituting the numbers, we find:

`V_s = V_p (N_s)/(N_p)=(120 V)(19)/(400)=5.7 V`