Question

A person is on the outer edge of a carousel with a radius of 20 feet that is rotating counterclockwise around a point that is centered at the origin. What is the exact value of the position of the rider after the carousel rotates 5pi/12 radians?

Answer 1

`\boxed{(5(-√(2)+√(6)),5(√(2)+√(6)))}`

Explanation:

The origin of the coordinate system is the center of the circle. So we have an angle that measures
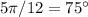`5\pi/12=75^(\circ)`. so the x-coordinate and y-coordinate can be found, by using trigonometry as follows:

`x=20cos(5\pi/12)=5√(6)-5√(2) \\ \\ Arranging:\\ \\x=5(-√(2)+√(6)) \\ \\y=20sin(5\pi/12)=5\sqrt(6)+5√(2) \\ \\ Arranging:\\ \\y=5(√(2)+√(6))`

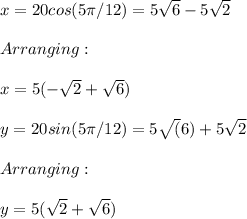
Finally, the exact value of the position of the rider after the carousel rotates
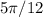`5\pi/12` radians is:

`\boxed{(5(-√(2)+√(6)),5(√(2)+√(6)))}`