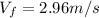Answer:
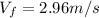
Firstly we have to draw the Free Body Diagram (FBD) as shown in the figure attached.
Where the weight
 of the block has an x-component and y-component:
of the block has an x-component and y-component:
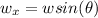 (1)
(1)
 (2)
(2)
As well as the Normal Force
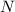 :
:
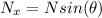 (3)
(3)
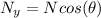 (4)
(4)
In addition, we know
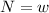 , then
, then

In the X-component:
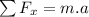
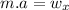 (5)
(5)
Substituting (1) in (5):
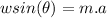 (6)
(6)
In addition, we know
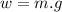 , where
, where
 is the mass of the block and
is the mass of the block and
 the gravity acceleration, which is equal to
the gravity acceleration, which is equal to
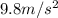
So:
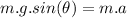 (7)
(7)
 (8)
(8)
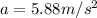 (9) >>>>This is the acceleration of the block
(9) >>>>This is the acceleration of the block
On the other hand, we have the following equation that expresses a relation between the distance
 with the acceleration
with the acceleration
 and time
and time
 :
:
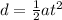 (10)
(10)
We already know the value of
 and calculated
and calculated
 , we have to find
, we have to find
 :
:
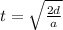 (11)
(11)
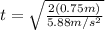 (12)
(12)
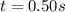 (13) >>>This is the time it takes to the block to go from the initial velocity
(13) >>>This is the time it takes to the block to go from the initial velocity
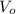 to its final velocity
to its final velocity
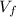
If the acceleration is the variation of the velocity in time, we can use the following equation to find
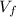 :
:
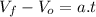 (13)
(13)
If

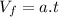 (14)
(14)
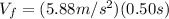 (15)
(15)
Finally we get the value of the Final Velocity of the block: