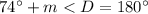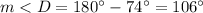Question

Can someone help me find this answer?

Answer 1

`m<D=106\°`

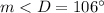
Explanation:

we know that

In a quadrilateral inscribed in a circle opposite angles are supplementary so

`m<A+m<C=180\°`

`m<B+m<D=180\°`

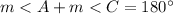
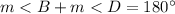
Step 1

Find the value of x

substitute the values

`x^(2) +3x=180\\ \\ x^(2) +3x-180=0`

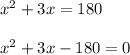
using a graphing tool

solve the quadratic equation

see the attached figure

`x=12\°`

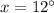
step 2

Find the measure of angle D

`m<B+m<D=180\°`

`m<B=7x-10=7(12)-10=74\°`

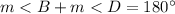
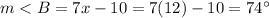
substitute

`74\°+m<D=180\°`

`m<D=180\°-74\°=106\°`