Question

What is the dimensional consistency of s = Vot + 1/2 at2​

Answer 1

The dimensional consistency of the equation s = Vot + 1/2 at2 can be determined by analyzing the dimensions of each term and checking if they match.

Step-by-step explanation:

The dimensional consistency of the equation s = Vot + 1/2 at2 can be determined by analyzing the dimensions of each term.

Using the given dimensions [s] = L, [v] = LT−¹, [a] = LT−², and [t] = T, we can substitute these dimensions into the equation:

[s] = [v] x [t] + 1/2 [a] [t]^2

L = (LT^(-1))(T) + 1/2 (LT^(-2)) (T^2)

L = L + L, which is dimensionally consistent.

Answer 2

The formula is dimensionally consistent

Step-by-step explanation:

Dimensional Analysis

There are three fundamental magnitudes in Physics:

L = Length

T = Time

M = Mass

All the formulas and equations that use physical magnitudes must be consistent in their units.

For example, the formula:

`\displaystyle s=v_ot+(1)/(2)at^2`

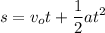
Is used to calculate the distance traveled by an object, knowing its initial speed vo, acceleration a, and time t.

The units for each magnitude are:

s = L

vo = L/T or
`LT^(-1)`

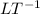
a = L/T^2 or
`LT^(-2)`

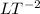
Let's analyze the dimensions of the magnitudes in the formula (the constant 1/2 is neglected because it doesn't affect the dimensional analysis):

`\displaystyle L=L/T*T+L/T^2*T^2`

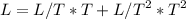
The first term simplifies to L because the T's simplify. The same happens with the second term, the squared T's simplify:

`\displaystyle L=L+L`

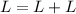
Thus the formula is dimensionally consistent