Question

In what ratio does the line x-y-2 = 0 divides the line segment joining (3, -1) and (8, 9)?

Answer 1

`3: 2`

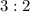
Step-by-step explanation

The equation of the line connecting (3,-1) and (8,9) is

`y - 2x = - 7...(1)`

The given line segment also have equation

`x - y - 2 = 0`

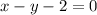
Or

`x - y = 2..(2)`

Adding equation (2) and (1) gives,

`- x = - 5`

`x = 5`

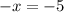
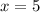
We substitute x=5 into equation (2) to get,

`5 - y = 2`

`y = 3`

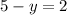
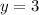
This means that the point of intersection is (5,3)

Let this point divide the line segment joining (3, -1) and (8, 9) in the ratio

m:n

Then,

`(3m + 8n)/(m + n) = 5`

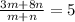
This implies that,

`5m + 5n = 3m + 8n`

`2m = 3n`

`(m)/(n) = (3)/(2)`

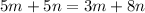
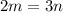
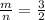
m:n=3:2