Question

Evaluate the integral. pls help me see the steps. thank you​

Answer 1

`-(2)/(15)(1-x)^(3)/(2)(3x+2)+C`

Explanation:

Use the method of integration by parts. The method is based in the following rule of derivation of two functions f and g :

`(fg)' = f'g+fg'\implies fg'=(fg)'-f'g\implies \\\int fg' dx = fg -\int f'g dx`

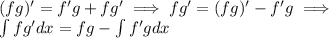
Identify suitable f and g in the original expression. I use:

`f=x\,\,\,, \,\,\,g=√(1-x)\\f'=1\,\,\,,\,\,\,g'=-(2)/(3)(1-x)^(3)/(2)`

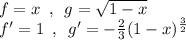
and write the integration by parts :

`\int x√(1-x) dx = -(2)/(3)x(1-x)^(3)/(2)+(2)/(3)\int (1-x)^(3)/(2)dx=\\ =-(2)/(3)x(1-x)^(3)/(2)-(4)/(15)(1-x)^(5)/(2)+C=\\=-(2)/(15)(1-x)^(3)/(2)(3x+2)+C`

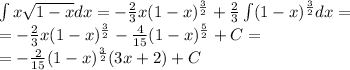
with C an arbitrary integration constant.