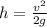Question

To apply the law of conservation of energy to an object launched upward in the gravitational field of the earth. In the absence of nonconservative forces such as friction and air resistance, the total mechanical energy in a closed system is conserved. This is one particular case of the law of conservation of energy. In this problem, you will apply the law of conservation of energy to different objects launched from the earth. The energy transformations that take place involve the object's kinetic energy K=(1/2)mv2 and its gravitational potential energy U=mgh. The law of conservation of energy for such cases implies that the sum of the object's kinetic energy and potential energy does not change with time. This idea can be expressed by the equation Ki+Ui=Kf+Uf , where "i" denotes the "initial" moment and "f" denotes the "final" moment. Since any two moments will work, the choice of the moments to consider is, technically, up to you. That choice, though, is usually suggested by the question posed in the problem. Using conservation of energy, find the maximum height h_max to which the object will rise

Answer 1

`h=(v^2)/(2g)`

Step-by-step explanation:

In absence of non-conservative forces, the mechanical energy of the object (sum of kinetic energy) is conserved:

`K_i + U_i = K_f + U_f`

where:

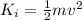`K_i = (1)/(2)mv^2` is the initial kinetic energy of the object, with mass m and launched with speed v upward

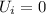`U_i = 0` is the initial potential energy of the object, which is zero since the object is launched from the ground

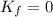`K_f = 0` is the kinetic energy of the object when it reaches its maximum height, and it is zero because at maximum height the speed is zero: v = 0

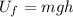`U_f = mgh` is the potential energy of the object at maximum height h, with g being the acceleration due to gravity

Therefore, the previous equation becomes

`(1)/(2)mv^2=mgh`

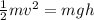
and by re-arranging it we find an expression for the maximum height:

`h=(v^2)/(2g)`