Question

If the slope is 3 what does it represent using this table

Answer 1

The table represents a linear function.

The graph of linear function y = 3x is attached below.

Explanation:

Given the table

Number of Hours 1 3 5

Number of Tents 3 9 15

Given that the slope is 3.

Finding the slope between (1, 3) and (3, 9)

Slope = (y₂-y₁) / (x₂-x₁)

= [9-3] / [3-1]

= 6 / 2

= 3

Finding the slope between (3, 9) and (5, 15)

Slope = (y₂-y₁) / (x₂-x₁)

= [15-9] / [5-3]

= 6 / 2

= 3

As the value of slope remains constant i.e. slope = m = 3.

Using the point-slope form of the line equation

`y-y_1=m\left(x-x_1\right)`

where m is the slope of the line and (x₁, y₁) is the point

substituting the values m = 3 and the point (1, 3)

`y - 3 = 3 (x-1)`

`y-3 = 3x-3`

`y = 3x-3+3`

`y = 3x`

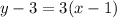
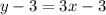
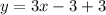
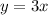
Comparing with the slope-intercept form of a linear function of line

y = mx+b where m is the slope and b is the y-intercept

slope = 3 and y-intercept b = 0

Therefore, the table represents a linear function.

The graph of linear function y = 3x is attached below.