Answer:
Approximately
 .
.
Step-by-step explanation:
Deduction of the formula
Let
 and
and
 denote the mass of the star and the planet, respectively.
denote the mass of the star and the planet, respectively.
Let
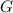 denote the constant of universal gravitation (
denote the constant of universal gravitation (
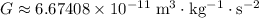 .)
.)
Let
 denote the orbital radius of this planet (assuming that
denote the orbital radius of this planet (assuming that
 is constant.) The question states that
is constant.) The question states that
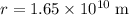 .
.
The size of gravitational attraction of the star on this planet would be:
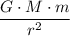 .
.
If attraction from the star is the only force on this planet, the net force on this planet would be
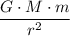 .
.
Let
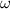 denote the angular velocity of this planet as it travels along its circular orbit around the star. The size of
denote the angular velocity of this planet as it travels along its circular orbit around the star. The size of
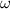 could be found from the period
could be found from the period
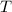 of each orbit:
of each orbit:
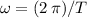 .
.
In other words, this planet of mass
 is in a circular motion with radius
is in a circular motion with radius
 and angular velocity
and angular velocity
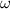 . Therefore, the net force on this planet should be equal to
. Therefore, the net force on this planet should be equal to
 .
.
Hence, there are two expressions for the net force on this planet:
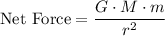 from universal gravitation, and
from universal gravitation, and
 from circular motion.
from circular motion.
Equate the right-hand side of these two equations:
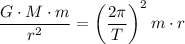 .
.
Simplify this equation and solve for
 , the mass of the star:
, the mass of the star:
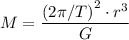 .
.
Notice that
 , the mass of the planet, was eliminated from the equation. That explains why this question could be solved without knowing the exact mass of the observed planet.
, the mass of the planet, was eliminated from the equation. That explains why this question could be solved without knowing the exact mass of the observed planet.
Actual Calculations
Convert the orbital period of this star to standard units:
 .
.
Calculate the mass of the star:
 .
.