Answer:
Explanation:
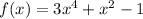
Let us do by trial and error method
f(0) = -1 and f(1) = 3
f(-1) = 3
i.e. f(1) = f(-1)
Since the function is a polynomial it is continuous.
This is an even function because f(x) = f(-x)
So if we find one root, we can easily guess the other root as -value
There is a root between 0 and 1 and also -1 and 0.
f(0.6) and f(0.7) have change of sign. SO root lies between 0,6 and 0.7
By filtering we find that the root is approximately 0,659.
BY symmetry about y axis, -0.659 is the other root
(x+0.659) and (x-0.659) are factors
When we divide by this product as x^2-0.434 we get quotient as
x^2+0,768
So the other two roots are imaginary.
Only real roots are
0,659 and -0,659