Question

(1) In the diagram below, AC || DF|| BH || and CB || FE.

Find four similar triangles Explain how you know they are all similar.

(2)Using the simillar triangles you found in 1. complete the following extended proportion. AB/AC=DE/?=?/DG=?/?

Answer 1

(1) Four similar triangles are: ABC, DBG, DEF, BEH

(2)
`(AB)/(AC)=(DE)/(DF)=(DB)/(DG)=(BE)/(BH)`

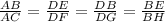
Explanation:

For part 1, you have to use the definition of the Side Splitter Theorem:

It's known that if a line is paralell to a side of a triangle and intersects the other two sides then the formed triangle is similar to the other because their sides are proportional

In the given diagram, the triangles ABC and DBG are similar because the line DG is paralell to AC. The same for DEF and BEH because DF|| BH and also for the other combinations of triangles.

For part 2, you have to apply the property of ratio:

The ratios of the lengths of the corresponding sides of similar triangles are equal. Therefore:

For the triangle DEF, the corresponding side missing is DF.

For the triangle DBG, the corresponding side missng is DB.

The missing ratio is the ratio of the triangle BEH, which is BE/BH

Answer 2

Part 1) Triangles ABC, DBG, DEF and BEH are similar

Part 2)
`(AB)/(AC)=(DE)/(DF)=(DB)/(DG)=(BE)/(BH)`

Explanation:

Part 1)

we know that

If two triangles are similar, then the ratio of its corresponding sides is equal and its corresponding angles are congruent

In this problem Triangles ABC, DBG, DEF and BEH are similar, because its corresponding angles are congruent by AA Similarity Theorem

Part 2)

Remember that

If two triangles are similar, then the ratio of its corresponding sides is equal

therefore

`(AB)/(AC)=(DE)/(DF)=(DB)/(DG)=(BE)/(BH)`