Question

The bids in an online auction are represented by the arithmetic sequence shown below. Write an explicit formula to represent the bids as an arithmetic sequence. What is the tenth bid?

Answer 1

Explanation:

Tenth bid is 300

Answer 2

Answer:
`A(n)=201+(n-1)11`

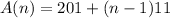
A(10)=300

Explanation:

The given arithmetic sequence : 201 , 212 , 223, 234,....

The first term = a =201

The common difference = d= 212-201= 223-212 = 234-223 =11

The explicit formula for arithmetic sequence is given by :-

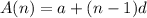`A(n)=a+(n-1)d` , where a is the first term and d is the common difference and n is the number of term.

Then for the explicit formula to represent the bids as an arithmetic sequence will be :_

`A(n)=201+(n-1)11`

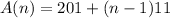
For n= 10

`A(10)=201+(10-1)11=201+(9)11\\\\=201+99=300`

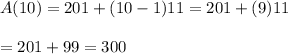
i.e. A(10)=300