Answer: h=7
Explanation:
By definition the function in vertex form is:
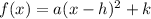
Where (h,k) is the vertex of the parabola.
You must group the terms that contain the same variable in the function:
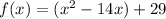
Complete the square as following:
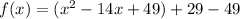
Now you must rewrite it as following:
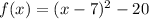
Then:
h=7