Question

Use the limit theorem and the properties of limits to find the limit.

Answer 1

Option B. ∞ is the correct option.

Explanation:

In this question the given expression for which we have to find the limit.

`\lim_(x\rightarrow \ \oe )(-7x^(3)+4x+1)/(-7x^(2)-9x+3)`

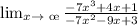
Now we will convert the expression as below

`=\lim_(x\rightarrow \ \oe )(-7+(4)/(x^(2))+(1)/(x^(3)))/(-(7)/(x)-(9)/(x^(2))+(3)/(x^(3)))`

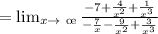
We have done this because we know
`\lim_(x\rightarrow \ \oe )(1)/(x)=0`

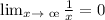
As we find the denominator as 0 therefore the limit of the given expression is ∞.

Answer 2

b. ∞

Explanation:

Given:

`\lim_(x \to \infty) ((-7x^3 + 4x + 1)/(-7x^2 -9x + 3))`

Here the highest degree of the denominator polynomial is 2. Therefore, divide both the numerator and the denominator by x^2, we get

`\lim_(x \to \infty) ((-7x +4/x +1/x^2)/(-7 -9/x + 3/x^2) )`

When we apply limit x -->∞, the numerator become -∞ and the denominator is -7.

Note: 1/∞ = 0

Therefore, we get

= (-∞ / -7)

= ∞ [Using the sign rule and dividing infinity by anything is infinity]

Answer: b. ∞

Hope this will helpful.

Thank you.