Question

True or False?
Picture provided below

Answer 1

The statement is true

Explanation:

To answer this question we must take the limit of f(x) when the function tends to 1. We must take this limit by the right and the left of 0.
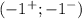`(-1 ^(+); -1 ^(-))`. If any of these limits tends to infinity then the function is discontinuous at x = -1.

But if the

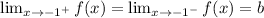`\lim_(x \to \\-1^+)f(x)=\lim_(x \to \\-1^-)f(x) = b` Where b is a real number

Then the function is continuous at x = -1

Then.

`\lim_(x \to \\-1^+)f(x) = \lim_(x \to \\-1^+)-2x +1\\\\\lim_(x \to \\-1^+)-2(-1) +1 = 3`

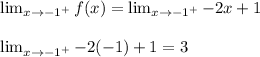
and

`\lim_(x \to \\-1^-)f(x) = \lim_(x \to \\-1^-) 2x+5\\\\\lim_(x \to \\-1^-) 2(-1)+5 = 3`

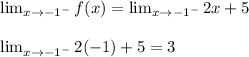
Then:

`\lim_(x \to \\-1^+)f(x) = \lim_(x \to \\-1^-)f(x) = 3`

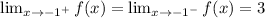
Therefore the function is continuous at x = -1. The statement is true