Question

A vehicle purchased for $22400 depreciates at a constant rate of 9%.

Determine the approximate value of the vehicle 13 years after purchase.

Round to the nearest whole number.

Answer 1

$15 827

Explanation:

The formula for the amount A of depreciation of an asset by an annual percentage rate is

A = V(1 - r)ⁿ

where

n = number of years

r = annual percentage rate

V = value

Data:

n = 13 yr

r = 9 %

V = $22 400

Calculations:

A = 22 400(1 - 0.09)¹³ =22 400(0.91)¹³ = 22 400 × 0.293 45 = $ 6573

So, the car lost $6573 in value because of depreciation.

Current value = 22 400 - 6573 = $15 827

Answer 2

The approximate value of the vehicle 13 years after purchase is $15827.

Explanation:

The formula for the value lost of an asset after n years, with depreciation rate r(in decimal) is given by:

`V = V_(0)(1-r)^(n)`

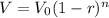
In which
`V_(0)` is the initial value.

In this problem, we have that:

A vehicle purchased for $22400 depreciates at a constant rate of 9%. This means that
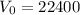`V_(0) = 22400` and
`r = 0.09`.

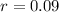Determine the approximate value of the vehicle 13 years after purchase.

This means that
`n = 13`.

So

`V = V_(0)(1-r)^(n)`

`V = 22400*(0.91)^(13)`

`V = 6573.34`

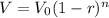
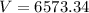
So, the approximate value of the vehicle 13 years after purchase is $22400 - $6573 = $15827.