Answer:
The area of the resulting figure is
 times smaller than the area of the original figure
times smaller than the area of the original figure
Explanation:
we know that
If two figures are similar, then the ratio of its areas is equal to the scale factor squared
I assume that the figure is a rectangle or a triangle
Let
z------> the scale factor
x-----> the area of the resulting figure
y----> the area of the original figure
so
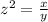
we have
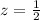
substitute
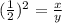
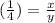
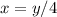
therefore
The area of the resulting figure is
 times smaller than the area of the original figure
times smaller than the area of the original figure