Question

A. A heat engine converts the flow of heat that results from a difference in temperatures into work. Write one or two sentences describing a heat engine that has a low efficiency by comparing the temperatures at which it operates.

b. How could you improve the efficiency of a heat engine by decreasing one of the temperatures at which the heat engine operates?

c. Calculate the amount of heat needed to warm 16.1 kg of water from a room temperature of 20.0°C to 60.0°C. Show your work.

d. A water heater has an efficiency of 67%. If the water heater takes in 6040 kJ of heat to heat up water for a dishwasher, how much total work does it provide? Show your work.

e. How much energy is lost to the surroundings while heating the water for one cycle of the dishwasher? Show your work.

Answer 1

a) Heat efficiency in terms of temperatures is

`\eta=1-T_2/T_1`,

where T1 - heater's temperature, T2 - cooler's one. We see that when T1=T2, efficiency is 0. The more the difference between the temperatures, the more efficient is the machine.

b) By decreasing T2. For example, on the whole vehicle's efficiency is higher in cold weather.

с) Q=cm(t2-t1)=4200*16.1*(60-20)=2704800 J.

d) Unfortunately, it provides less total work than it takes:

Q=W*0.67=6040*0.67=4046.8 kJ

e) Lost = Consumed - useful = 6040-4046.8=1993.2 kJ.

Answer 2

PART A)

efficiency of the heat engine is defined as

`Efficiency = (W_(out))/(Q_(in))`

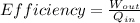
So it is ratio of output work and input Heat energy

So here we can say that

In order to increase the efficiency we have to increase the output work with less amount of input heat

this is related to temperature as

`efficiency =1 - (T_2)/(T_1)`

now we can say that in order to increase efficiency T2 must have to decrease and T1 have to increase

Part b)

now we can say that in order to increase efficiency T2 must have to decrease and T1 have to increase

So on decreasing the temperature T2 and increasing the temperature T1 we will get more efficiency of heat engine

Part c)

heat require to increase the temperature

`Q = ms\Delta T`

now we have

m = 16.1 kg

s = 4186 J/kg C

`\Delta T = 60 - 20 = 40^oC`

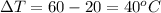
now we have

`Q = (16.1)(4186)(40) = 2.69* 10^6 J`

Part d)

Heat taken by heater = 6040 kJ

efficiency = 67%

now we have

`efficiency = (W)/(Q)`

`0.67 = (w)/(6040 kJ)`

`W = 4046.8 kJ`

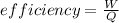
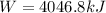
Part e)

Energy lost to the surrounding = Heat given - Work done

Energy lost = 6040 kJ - 4046.8 kJ

Energy lost = 1993.2 kJ