Question

Analyze the zeros of f(x)=x^4-3x^3-2x^2+3x-5

Determine the number of complex zeros.


a.2

c.1

b.4

d.3

Answer 1

a. 2

Explanation:

The given function is

`f(x)=x^4-3x^3-2x^2+3x-5`.

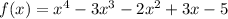We can see from the graph that the graph has two x-intercepts.

This means that the function has two real zeros.

By the fundamental theorem of Algebra, the function is supposed have four roots since it is a polynomial of degree 4.

Therefore, the other two zeros are complex.

See graph