Question

Sarah invested $38,700 in an account that paid 6.2% annual interest, compounding monthly. She left the money in this account, collecting interest for three full years. Approximately how much interest did she earn in the last month of this period?

Answer 1

Answer: Thus interest earned in the last month of this period is 232.20 $.

Step-by-step explanation:

Principal (P) = 38700

Annual rate of interest = 6.2 %

Monthly rate of interset (R) =
`(6.2)/(12)=0.52`

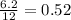
Time = 3 years = 36 months ( 1 year = 12 months )

Interest earned in n months =
`P(1+(R)/(100))^n-P`

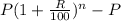
Interest earned in the last month of 3 years = interest earned in 36 months - Interest earned in 35 months =
`[38700(1+(0.52)/(100))^(36)]-[38700(1+(0.52)/(100))^(35)]=38700(1.205-1.199)=232.20`

Thus interest earned in the last month of this period is 232.20 $.